In Mild Praise of John Conway
(And Peter Alfeld)
(And Matt Samore)
My favorite university teachers have been mathematicians.1
This might make me an anomaly.
And this is not to say that I’ve enjoyed all my mathematics professors. Reflecting back, I can’t think of any mathematics professors that were really poor, although a couple of my math professors impacted me negatively on my career goals but this was in exchanges outside the classroom.
I’ve long been under the spell of Peter Alfeld, from whom I took a year-long applied mathematics series when I was an electrical engineering undergraduate at the University of Utah. I always tell people that Peter was my favorite professor, ever. I try to emulate Peter’s teaching style as much as I can. (Although I’ve always stuck to LaTex, never learning to use plain Tex.) I have multiple books on my bookshelves that I can trace directly back to Peter, most importantly Richard Westfall’s Never at Rest, Courant and Robbins’ What is Mathematics?, and a now well-worn VNR Concise Encyclopedia of Mathematics. I wish I had taken more classes from Peter. Perhaps my favorite mathematics topic was complex analysis which I had both as an undergraduate from a visiting professor from Germany that I rather enjoyed, and again as a graduate student at the University of Wisconsin, from an affable professor who, when he was hopelessly lost in a derivation, would change course and talk about the history of mathematics. His favorite mathematician was Apollonius of Perga. Outside of the classroom, encounters with mathematicians have been equally enriching. While at Utah as a faculty member, I had the pleasure of working some with the eminently quotable Fred Adler.2 My favorite non-classroom lecture I ever attended was a Frontiers of Science lecture given at the University of Utah by the mathematician John Conway.
Thanks to a website created by—Peter Alfeld!—I know that lecture, entitled “Knots and Numbers, Tangles and Bangles,” was given on May 15, 1996, when I was at the University of Utah studying medical informatics and still wishing I hadn’t been scared away from pursuing a PhD in applied mathematics. My memories of the talk have long since faded, other than that Conway was very dynamic and that he got a subset of the audience tangled up in knots on the Fine Arts Auditorium stage. Thankfully, he gave a similar talk at Berkley around the same time and a poor quality video of the talk can be seen here on YouTube. (Was he more sedate in a formal mathematics colloquium?)
Sadly, Conway died from Covid 19 early this spring. Years ago I bought Conway’s The Book of Numbers which I have always enjoyed, although I have been frustrated that understanding didn’t come quite as easily as enjoyment. Prompted by his death, I recently started reading Conway’s biography, Genius at Play, written by the science writer Siobhan Roberts. (I was also prompted to start reading the biography when my son had to program the Game of Life for his Haskell class last term, although I’ve read enough of the biography to guess that Conway might cringe in his grave thinking that that might have prompted me to read the book.)
Since I’ve just wrapped up teaching my second class at the University of Melbourne, both online due to Covid-19, I was rather struck by the book’s description of Conway’s teaching style.
I wanted to share a couple of long excerpts with my fellow teachers for (hopefully) inspiration.
In the book Roberts puts direct quotes from Conway in bold. I’ve replicated that here.
During an interview in a student publication, the Princeton Eclectic, an admiring reporter noted, “You’re renowned for your energetic and slightly crazy teaching style. . . . Where does your teaching style come from?”
Deep inside.
Some might just call it crazy, plain and simple.
But with respect to teaching, it’s not just for the sake of being crazy. It really does help people think. I mean one of the tricks I do, which I only started really when I came to Princeton, is shouting. There was this time when I was conducting this geometry course, and the kids always forgot one particular point, which was that the gyration point was not on a mirror. So one time I said, “A GYRATION POINT IS NOT ON A MIRROR”—I shouted it like all hell. And every now and then we’d organize shouting competitions with the whole class: “The gyration point is a point . . . that is NOT on a mirror!” Everybody would shout it. Actually once I shouted so loud that my trousers split. I sort of jumped up into the air at the shout. I jumped up like a . . . I don’t know, like a cartoon figure so to speak. Legs apart and everything and this great ripping sound . . .
So, the reporter asked, these techniques help induce understanding?
Well, I don’t know. Sometimes they just wake people up.
(Roberts, Siobhan. Genius At Play (p. 285). Bloomsbury Publishing. Kindle Edition.)
Conway adores naming and renaming things, and renaming things that he named, or renamed, again and again and again….”That’s part of his magic,”” says [Bill] Thurston. “He thinks a lot about how people will understand something, he thinks a lot about ways to communicate with people, to surprise and impress, not to keep them mystified, but to make them wake up and take note.”
Thurston was also known for his opinions on mathematics education. In his view, students did not benefit from the first-year linear algebra hurdle and the 10 varieties of calculus thereafter. An egalitarian-minded Quaker, at Princeton he created a new kind of math course, a course that would appeal to math and poetry majors alike. He named it “Geometry and the Imagination,” inspired by the book of the same name published in 1932 by the universalist mathematician David Hilbert. (Hilbert, upon hearing that a student had dropped math to study poetry, said, “Good! He did not have enough imagination to become a mathematician.”) To publicize the new course, Thurston and his co-organizer Peter Doyle, then Thurston’s postdoc student and now a professor at Dartmouth, placed ads in the university’s student newspaper, the Daily Princetonian. Thurston stressed that this “creative mathematics” would still involve serious hard work, but curiosity-driven fun would be paramount; usually in mathematics there was too much delayed gratification. They expected 20 or so takers. When the course drew 92 students—the kind of number usually reserved for the no-brainer gut courses—they roped in the new man Conway, the perfect addition to their sideways, subversive effort. Together, the Thurston-Doyle-Conway trio designed a course tackling the most intractable of mathematics problems: How to teach.
For starters, the professors made a ritual of entering the classroom en masse, sometimes with great pomp and circumstance, sometimes carrying a flag, sometimes wearing bicycle helmets, often pulling a red kiddy wagon heaped with polyhedra, mirrors, flashlights, and fresh produce from the grocery store. In Thurston, Conway found a true soul mate, because both men loved lecturing with vegetables (Thurston was a goody-goody vegetarian and christened all the computers in Fine Hall with vegetative names). In contemplating the curvature of surfaces, for instance, Thurston and Conway might lead the class in peeling potatoes—peeling a single strip around the potato’s equator and laying the strip flat on the blackboard in order to measure the angle by which the peel fell short of closing a full circle, or the angle by which it exceeded. This, they explained, measured the Gaussian curvature, the total curvature, for the region of the potato enclosed by the peel. To the same end, Thurston liked to get the class cutting up lettuce, cabbage, and kale. Conway, meanwhile, not a vegetarian, preferred using as a prop his protuberant stomach. In this display of curvature he turned down the lights, laid himself on a table before the class, lifted up his T-shirt revealing his magnificence, and planted the back end of a shining flashlight on his belly button. Tracing the flashlight around his stomach’s circumference, he advised the students to watch the path the light beam traveled on the ceiling and to imagine how the light’s path might differ were it traversing flatter terrain.
Another exercise explained the bicycle helmets. Prior to class the teachers found large rolls of paper, tore off strips that were 6 feet by 20 feet at least, and borrowed some bicycles. They painted the tires of each a different color, and then starting from some small distance away, rode a bike across a strip of paper. In class, the students were presented with this artwork and asked, regarding each set of tracks, “Which way did the bicycle go?”
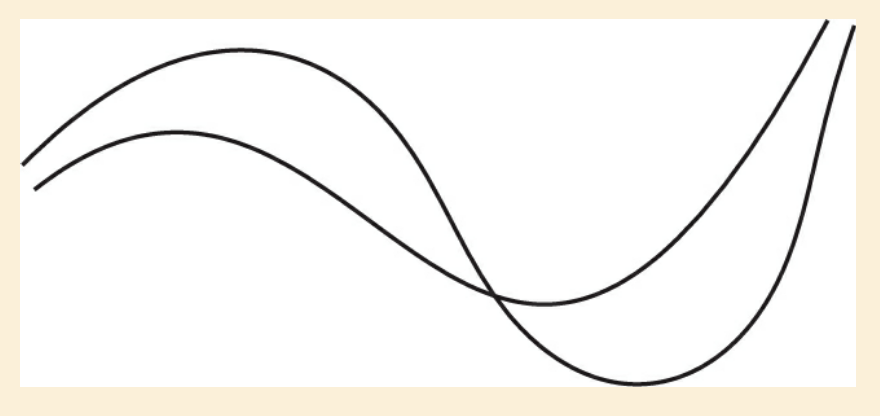
The first step is identifying which track was made by the back wheel and which by the front. There are many ways to do it, but the easiest is to realize that the front wheel’s track always has more pronounced curvature, initiating turns this way and that, and then pulling the back wheel along on a smoother path.

Determining the direction of travel then involves taking a tangent line to the back wheel’s track (the bold track in the diagram above) and marking where it intersects the front wheel’s track, producing tangent line segments.

When considering the tracks and the direction of the bike’s travel, these tangent segments should equal the length of the bicycle as measured between the centers of the front and back wheels. Measuring the tracks in one direction, the tangent line segments will be of equal or constant length, while in the other direction the tangent line segments will be of different lengths. So in determining the direction of travel, the teachers advised students to weigh the hypothesis that a bicycle of constant length went one direction against the hypothesis that a bicycle of variable length went the other way (moving to the left, $T_1L_1\ne T_2L_2$, but moving to the right, $T_1R_1=T_2R_2$, therefore the bike was traveling to the right).
A certain set of tracks, however, stumped the students. For that set Peter Doyle had pedaled up the sheet of paper and then back again, but on a unicycle.
All in all, the course was a big exercise in imagining, drawing heavily on symmetry and geometry. “Geometry is the user-friendly interface of math,”” as Thurston liked to say. The modus operandi was to expose the students to a barrage of activities that would change the way they saw and thought about things, mathematical things at least. Usually, as Conway commented to a reporter, teaching geometry from a textbook is like taking a dog for a walk around the block:
You take the student out in the yard and let him do his thing, then you bring him back in.
“Geometry and the Imagination,”” by comparison, let students loose to romp and run free.
You have to let them exercise.
Roberts, Siobhan. Genius At Play (pp. 292-295). Bloomsbury Publishing. Kindle Edition.
[Due to insomnia and other emotional and health issues Conway’s] daytime performance suffered, however, and during a games conference in Berkeley in July 1994, he gave a really rotten showing.
It was a disaster. On the other hand, a talk that I think was a disaster other people often think was quite reasonable. I’m so much better at giving talks than other people that . . . well, never mind. But it really is the case. The prevailing standard in mathematics is just god-awful. But anyway, I gave my talk and it wasn’t much good, that’s all you need to know.
The next day there was a no-show, and the conference chair, Richard Guy, then working with Conway on The Book of Numbers, asked his coauthor to fill in. Conway gave his usual knee-jerk response in the affirmative. But given the recent disaster he immediately had second thoughts. He asked Guy when this talk was scheduled. “Now!” Walking the short distance to the front of the lecture room, Conway did his typical impromptu preparation and decided it would be a good idea to pull out his golden oldie, “The Lexicode Theorem—Or Is It?” This talk went over less disastrously, in Conway’s estimation; it might even have been something of a success. As the audience milled around afterward, gradually making their way out of the room, a bottleneck formed at the exit and Conway ended up behind the door waiting to leave. As he stood there unseen by the people filing out, he heard the Canadian mathematician Aiden Bruen give his assessment: “That’s more like the Conway we know and love.”
That told me 2 wonderful things: That my impression of the first talk was right—I had an objective opinion, someone else thought the first talk was a disaster too, which I needed to know because I needed to know that my impressions were in accordance with reality. And then it told me that the second talk was, in fact, really more like the Conway we know and love.
(Roberts, Siobhan. Genius At Play (pp. 320-321). Bloomsbury Publishing. Kindle Edition.)
What does this have to do with my life?
I’m struck by how much Conway loved play and wanted the students to play, versus being walked around the block like a dog on a lead. This is similar to what my mentor Matt Samore advocated in a recent interview I did with him:
I remember a lot of discussions with Charlene Weir, one of our professors in biomedical informatics who is also a psychologist, who talked about the benefit of giving people tools that they can play with, the benefit of exploration.
I think a lot about making interactive learning tools for students, but I wonder to what extent these tools are things the students actually want to play with? (Should I call them toys rather than tools?) And how do I draw students to the toy-tools so that they want to explore?
Just yesterday I told my department chair that my goal in the medical school was to “Empower medical students to incorporate digital tools to deliver more humane and personalized care to their patients” and I would achieve this in part by “Creating engaging yet brief learning materials reviewing historical co-evolution of medicine and technology.” I suspect that like mathematics lectures, “the prevailing standard” in much of computing and informatics (and medicine?)—including my own—“is just god-awful.”
In front of the class, I can be kind of a performer. My wife used to laugh at the Elvis-like gyrations of my hips as I taught magnetic resonance imaging and I can handle a microphone in my class like Phil Donahue, running from student to student to get their thoughts and feelings. I can weave David Bowie (“Rebel, Rebel” as the theme song for informatics) and the Specials (“Where did you get that blank expression on your face” when I teach regular expressions) into a lecture.
But online?
What is the equivalent of having group yelling sessions, marching tours to stare at bricks or group knot tying (as shown in the Berkley video)? Perhaps more importantly, when you are not the John von Neumann Professor of Mathematics at Princeton, a fellow of both the American Academy of Arts and Sciences and the Royal Society, the illustrious creator of the Game of Life, discoverer of the surreal numbers, and the Monster Group, can you get away with jumping, yelling, and ripping your pants?
Should I try to find out? At least without the ripping pants?
1 I have to throw the university qualifier in because my favorite high school teacher was Bill Laursen, my art teacher, who might be an adept mathematician but I don’t know for sure.↩
2 I did briefly sit in on one of Fred’s classes (mathematics of cancer) where I learned one of his teaching techniques: a group rage against an equation.↩